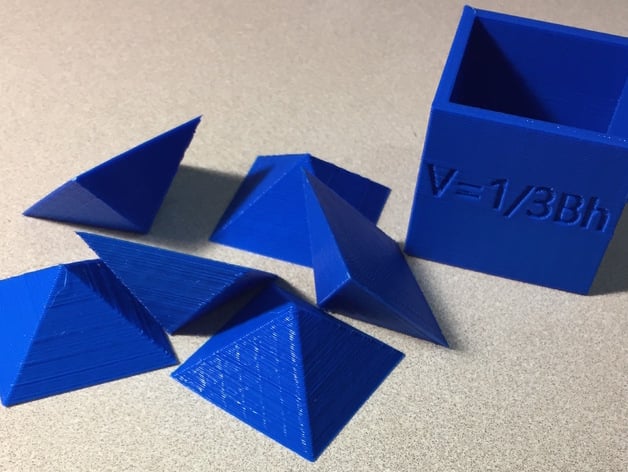
Visual Proof of Volume of a Pyramid

thingiverse

Another proof of volume of the pyramid, using 6 pyramids with the height half of that of the prism with the same base. Consider the height of the cube is 2x B = (2x)^2 = base area V = volume of each pyramid 6V = cube volume V = 1/6(2x)^3 = 1/6(2x)(2x)^2 = 1/3x(2x)^2 thus V = 1/3 (B)h, where h = height of the prism