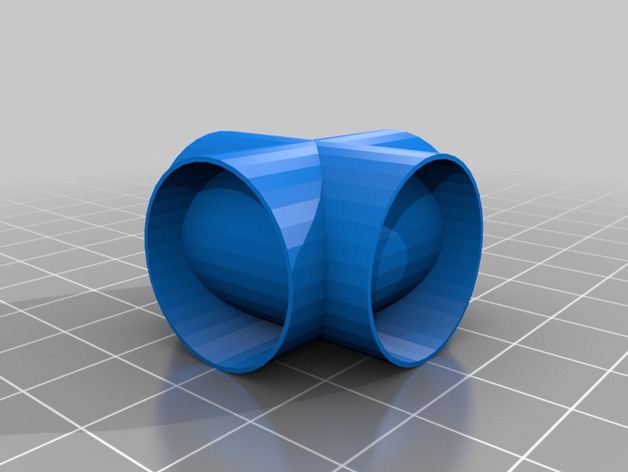
Two Intersecting Cylinders

thingiverse

The Steinmetz Solid: A Mathematical Marvel Imagine two cylinders, one inscribed within the other, their circular bases intersecting in a most intriguing way. This configuration is known as the Steinmetz solid, named after the mathematician Simon A. Stevin (1548-1620) who first described it. The two cylinders are positioned such that their axes coincide at a single point, allowing their circular bases to overlap and form a shape unlike any other. The resulting intersection creates a three-dimensional space that is both fascinating and challenging to analyze. Mathematicians have long been fascinated by the Steinmetz solid's unique properties, which defy easy description. Its surface area is comprised of two distinct regions: a spherical cap at one end and a cylindrical surface at the other. But what makes this shape truly remarkable is its inability to be dissected into simpler shapes using only straight lines. The Steinmetz solid has captivated mathematicians for centuries with its intricate geometry and counterintuitive behavior. Its study has led to breakthroughs in fields ranging from calculus to topology, inspiring new approaches to problem-solving and theoretical mathematics. One of the most striking aspects of the Steinmetz solid is its relationship to the famous Kepler-Poinsot polyhedra. These highly symmetrical solids have been a subject of fascination for mathematicians and scientists since the 16th century, and the Steinmetz solid's connection to them has shed new light on their properties. In recent years, advances in computer-aided design (CAD) technology have made it possible to visualize and manipulate the Steinmetz solid with unprecedented precision. This has allowed researchers to explore its behavior under various conditions, pushing the boundaries of our understanding of this enigmatic shape. The Steinmetz solid remains an active area of research, with new discoveries and insights continually emerging. Its study continues to inspire mathematicians, scientists, and engineers alike, driving innovation and advancing our knowledge of the intricate relationships between geometry, topology, and calculus.