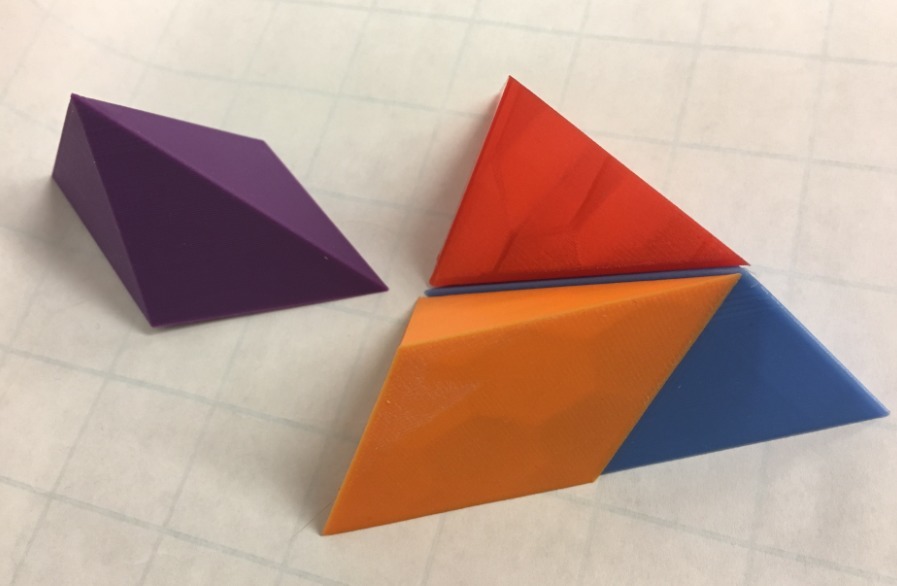
Tetrahedron, Puzzle, Triangular Pyramid, Dissection, Four Pentahedra

thingiverse

Dissecting a Regular Tetrahedron into Four Congruent Pentahedra The regular tetrahedron, a Platonic solid, can be dissected in multiple ways to create a puzzle. In this project, a tetrahedron is sliced into four congruent pieces using two planes that contain the midpoints of three edges (see figure below). Each piece forms a pentahedron with two equilateral triangles, two right triangles, and a rhombus. To assemble a complete tetrahedral puzzle, you will need 4 copies. This project can serve as an entry point for learning about 3D sketching and extruding at a taper angle. The Dihedral angle of a regular tetrahedron is arccos (1/3). It's also worth noting that a tetrahedron can be constructed by slicing a cube. This provides an opportunity to explore different methods of creating three-dimensional shapes. Cundy, H. M., & Rollett, A. P. (1961). Mathematical models. London: Oxford University Press. (p. 202). The authors provided a net but did not reveal the method for slicing a tetrahedron into four congruent pieces.