Solid of revolution

thingiverse

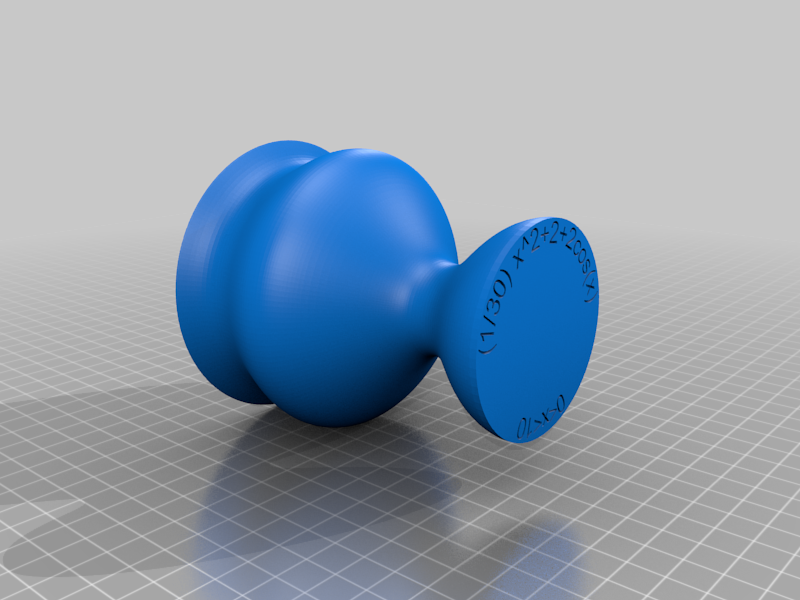
These four vases (solids of revolution) all have the inner volume equal to the solid of revolution they are based on. The dimentions are given in cm. Use these as an exercise in math class by calculating the volume of the solids, and testing it afterwards. The volumes are all challenging to solve by hand. They are a good way to illustrate the usefulness computer calculations for volume calculations. The function of each of the solids, as well as the range of x-values are printed in the bottom of the vases. I've considered these four vases to be four different difficulty grades: (easy) Tricky, Vase 1 (medium) Taxing, Vase 2 (medium/hard) Tough, Vase 3 (hard) Tenacious, Vase 4 Vase1: 0.3x+2, x∈[0, 10] Vase2: 1/30 x^2+2, x∈[0, 10] Vase3: 3+2⋅1.2^(-x)⋅cos⁡(x), x∈[0, 10] Vase4: 1/30 x^2+2+2 cos⁡(x), x∈[0, 10] I've only tested these once, and the result was not 100% equal to the calculated volume (I measured by filling them with water, and measuring the volume of the water afterwards. A more precise measuring method could be measuring the weight of the vases before and after they are filled). They *should* however be correct. The reason the models are slightly taller than 10cm, is because 10cm is the inner height of the vase.