Riemann Surface of R^1/3

thingiverse

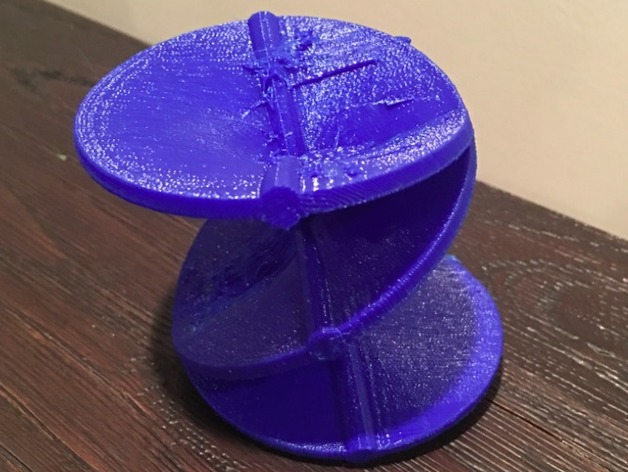
Object: Riemann Surface of function f(z)= z^(1/3) Created By: Hope Roberts Date, School & Place: 3/04/2016 George Mason University located in Fairfax, Va. The creation took place in Mathematica for a class project for Math 493, Math Through 3D Printing. Background of Riemann Surfaces Riemann surfaces are named after Bernhard Riemann who was the first to study them. Riemann surfaces are one way to show multi-valued functions. Showing that an element from the domain can map to multiple places in the codomain. They resemble surface-like structures consisting of infinite sheets that are separated by vertical distance. They are configured in the complex plane, which has vectors 1 and i where i is imaginary, and these vectors span the complex numbers. All complex numbers match to unique points in the complex plane (mathworld). Another way to show multi-valued functions is branch cuts, which is to take lines or line-segments of the multi-valued functions. Representing a multivalued function is to ascribe not one point but instead infinite points to the domain. The sheets represented by the mappings from the origin are all interconnected because it is undefined at the origin, which makes the origin, a point not part of the domain. The functions can be oriented in different ways by multiplying by the complex number. My function used for the Riemann surface was z^(1/3) and the surface was mapped using polar coordinates with the branches of the surface the tubes on top. The branches also called branch cuts that are on my surface representing where the function is not continuous and is also non-differentiable. The branches show when a sheet of the surface connects another sheet of the surface, which can be seen by the changing colors in the surface in the Mathematica code. As you increased distance in the polar coordinates, you continue crossing branch cuts where the sheets are meeting. This is seen in my code and in my print represented by the tube overlay. The second picture shows the surface without the tube overlay. The third picture shows the tubes separate. The fourth shows the combination. And the final picture shows the STL file loaded onto MakerBot. Print Settings Printer Brand: MakerBot Printer: MakerBot Replicator (5th Generation) Rafts: No Supports: Yes Resolution: 0.30mm Infill: 10% Notes: Instructions for Printing: In MakerBot on the 5th, I rotated the object until it was in the most flat position making sure to lay flat and on surface of the platform. I then increased the size to an approximate 4-hour print time which was approximately 70mm in the x direction, 72 mm in the y-direction, and 73 mm in the z direction. Rafts were taken off, and supports were left on. The support settings were adjusted so that the support layers were less thick so that taking off the support would be easier and less likely to break the structure. Post-Printing Riemann Surface showing branches where function is undefined closer view of Riemann Surface How I Designed This of Creation and Equations Used: While searching for information on my function, I discovered framework coding on the same function in Mathematica by Shutao Tang. From Shuao Tang’s code, I edited the following; The parametric plot 3D command; I added thickness command to thicken the overall surface for printing. The Evaluate command; I took out absolute thickness command replacing with the tube command in order to create tubes with assigned values that would map on the surface where the function was discontinuous. I also changed the range of the tube to match the surface when placed on top. Putting the tubes and the function together, insuring the tubes were large enough to be seen on the surface, I exported adding file to MakerBot. The following Equations were used or found helpful for this project; f(z)= z^1/3 where z=x+iy=r*i*θ in polar coordinates Riemann Approximation: ∑ from n to (k-1) with (f_(x_k) )∆xk where ∆(x_k)→0 Limit is known as Riemann integral of f(x) over closed interval [a,b]. (mathworld) Mesh size → Given by closed interval [a,b] partitioned by points