Riemann Surface in Polar Coordinates

thingiverse

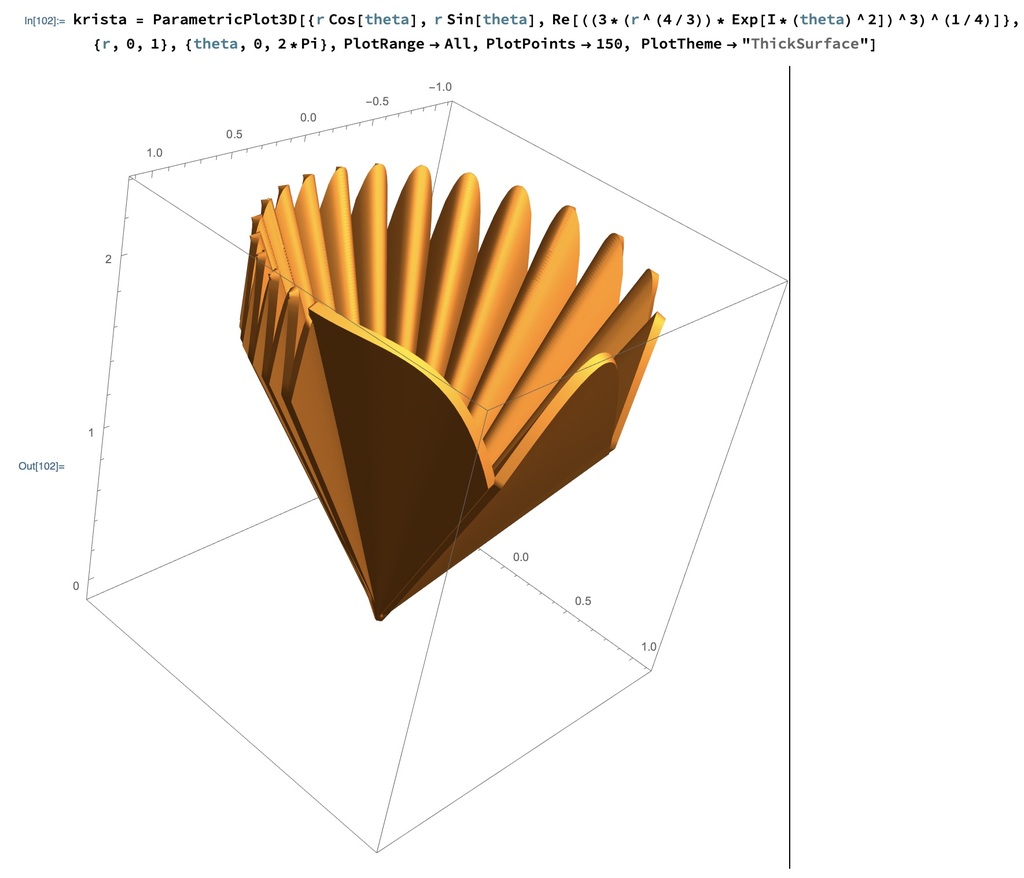
Riemann Surface in Polar Coordinates Krista Cimbalista 12/6/2020 GMU MATH 401: Mathematics Through 3D Printing Riemann surfaces are functions that take in curved surfaces as their domain. They output functions that are both one-to-one and continuous. Often, they are helpful for visualizing functions that are of four dimensions [1] I coded this function by taking an example from the notebook provided to us in class called “riemannsurface.nb” and changing the given values until I got a result that I liked. The first image is a picture of the original from the notebook provided to us. The second image is my first creation. This version had a couple of problems, as it was not defined in exactly the way it needed to be. The final version I created is more correct for this assignment than my previous attempts. The third and fourth images are pictures of the real and imaginary parts of my function for this project, respectively. My equation for this version is z = (27^.25)(r)(e^[.75i(theta)^2]). The parameters used were theta between 0 and 2pi and r between 0 and 1. It is easy to change this look of this plot by only changing the values in the equation. For example, change (27^.25) to 1. This results in a vertically squished version. As another example, change the power from ¼ to ⅓ and you get taller rims around the top. Finally change theta^2 to theta and you get a three-sided shape. This is a process similar to what I did to create this plot from the original. [1] https://www.youtube.com/watch?v=4MmSZrAlqKc&list=PLiaHhY2iBX9g6KIvZ_703G3KJXapKkNaF&index=13&ab_channel=WelchLabs