Riemann surface Arcsin

thingiverse

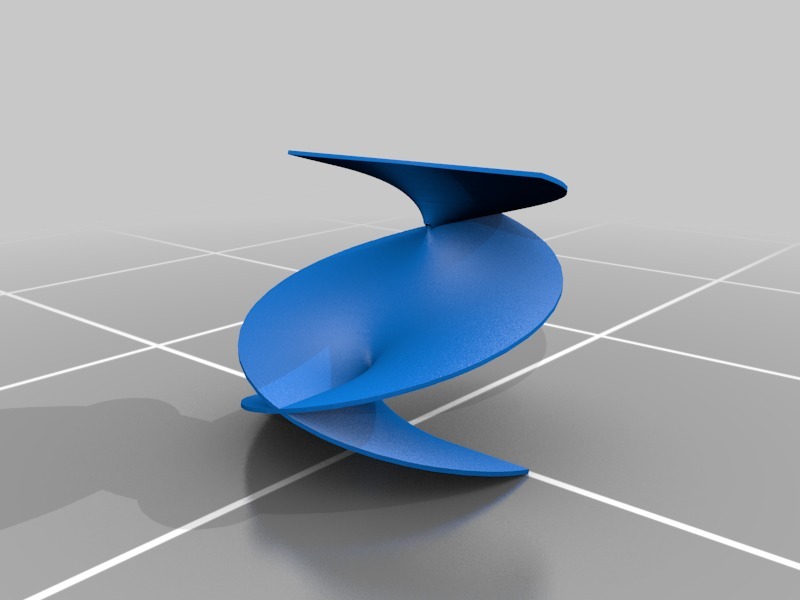
The Riemann Surface of Complex Function Arcsin(Z) The Arcsin(z) complex function has a particularly fascinating topological structure, manifesting as an infinite sheeted Riemann surface. This intricate geometry arises due to the multiple-valued nature of the inverse sine function in the complex plane. Visualizing the Arcsin(z) function, we observe that it branches out infinitely, resembling a tree with an endless number of leaves. Each leaf represents a distinct branch of the function, corresponding to different values of the argument z. As z varies across the complex plane, each branch intersects with adjacent ones, forming an intricate network. This Riemann surface is not simply connected, meaning it has "holes" or "tunnels" that allow for non-contractible loops. In other words, it's impossible to shrink any closed curve on this surface to a point without intersecting itself. This property gives rise to the rich geometric and analytic properties of the Arcsin(z) function. The Riemann surface of Arcsin(z) can be understood as a two-dimensional manifold with an infinite number of sheets, each corresponding to a specific branch of the function. By navigating this complex topological landscape, mathematicians have gained valuable insights into the behavior of complex functions and their singularities.