Pythagorean Theorem Proof

prusaprinters

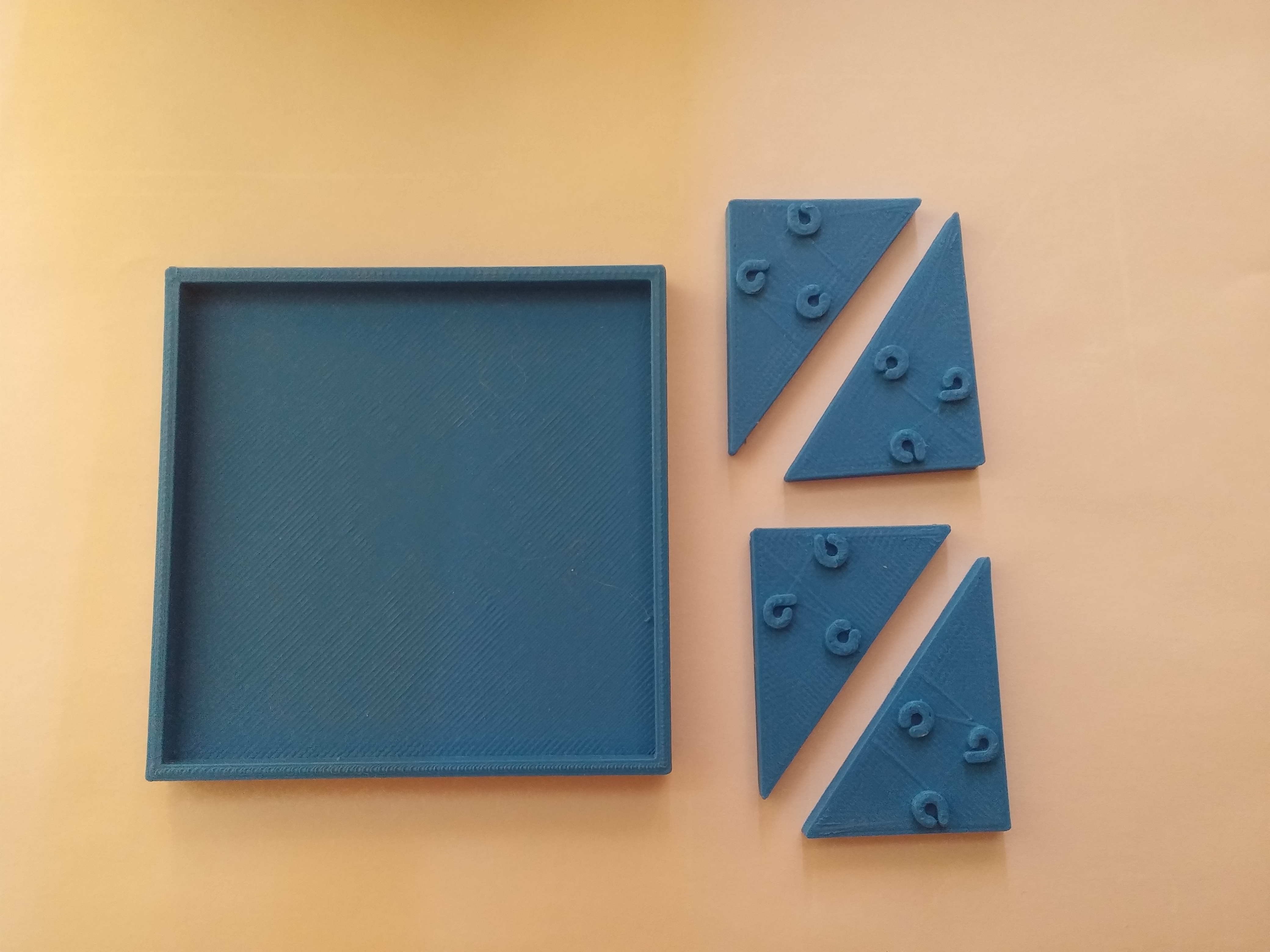
<p>This is a simple manipulative that shows how the Pythagorean Theorem can be proven using rearrangement.</p><p>We start with a tray with a fixed internal area equal to (a+b) squared and four identical triangles with sides of length a, b and c.</p><p>If we arrange the triangles around the perimeter of the tray, as shown in figure one, the negative space (space not occupied by the triangles) is a square with sides of length c.</p><p>When we rearrange the triangles to form the rectangles, as shown in figure two, the negative space is split between two squares, one with sides of length "a" and a second with sides of length “b.”</p><p>Because the negative space cannot change, this proves that a2 + b2 = c2.</p><p>This thing was made with Tinkercad. Edit it online <a href="https://www.tinkercad.com/things/8ba9Bfx64fZ">https://www.tinkercad.com/things/8ba9Bfx64fZ</a></p><p>&nbsp;</p><p>Originally posted on Thingiverse.</p>