Pythagorean Theorem on Hinges with Equilateral Triangles
thingiverse
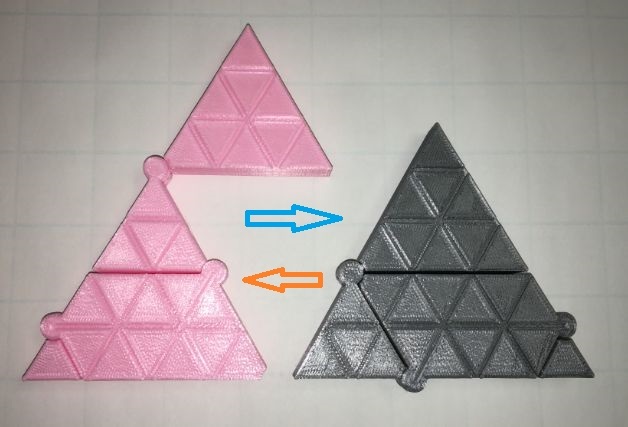
####Pythagorean Theorem, Equilateral Triangles, Math Puzzles ##### Can an equilateral triangle be dissected and turned around on the hinges into two equilateral triangles? How about the other way around? The Pythagorean Theorem is typically introduced using squares on the three sides of a right triangle. It is fine! However, we don’t have to use squares. Any similar 2D shapes constructed on the three sides of the right triangle will do the job for establishing the Pythagorean Theorem. Equilateral triangles (and semicircles) are quite interesting shapes to use in K-12 math classes. The present design uses *equilateral triangles* with three hinges to demonstrate the fact that a 3-equilateral triangle and a 4-equilateral triangle can be assembled into a 5-3-equilateral triangle. One can use two sets of equilateral triangles to show the relationship. In essence, we are using 1-equilateral triangles to measure areas. The bottom line is that all equilateral (and all semicircles) are similar to each other. This design also works like a math puzzle without mentioning the Pythagorean Theorem. ####Among the Files 1. Right triangle plus a set of equilateral triangles 2. One set of equilateral triangles without the right triangle Have fun playing with math ideas!
With this file you will be able to print Pythagorean Theorem on Hinges with Equilateral Triangles with your 3D printer. Click on the button and save the file on your computer to work, edit or customize your design. You can also find more 3D designs for printers on Pythagorean Theorem on Hinges with Equilateral Triangles.
