Project 9 Riemann Surface

thingiverse

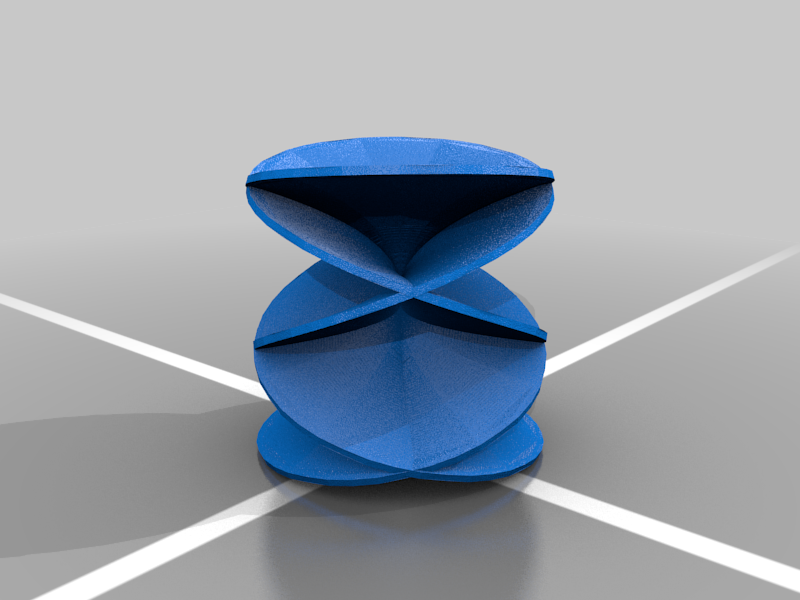
Project 9 Riemann Surfaces 12/3/21 Austin Fletcher George Mason University Math 401 Mathematics Through 3D Printing This print is a visualization of a Riemann surface. It represents {rCos[Ø], rSin[Ø], Im[(r)^(2/5)e(I Ø(2/5)]. Where r goes from 0-1 and Ø goes from 0-10π. I took my inspiration from this in the professors examples. I was able to take one of the examples and change the exponent on r to add more twists and complexity to it which I found to look interesting. This specifically shows the imaginary part but the only difference between the real and imaginary is the shape simple turns slightly. Once I had this shape designed, I had to scale it by 4000% in the slicer because it was so small and then I printed it on the Ultimaker S5 with green Tough PLA filament. Patrick advised that I do 18% infill and use tree supports for this print and it was estimated to take roughly 4 hours. Since this was the last week and I print on Thursdays, I was not able to make it back to get a picture of my actual object so you can see the object in the stl and mathematica notebook screen shots.