Polypanel Penrose Puzzle

myminifactory

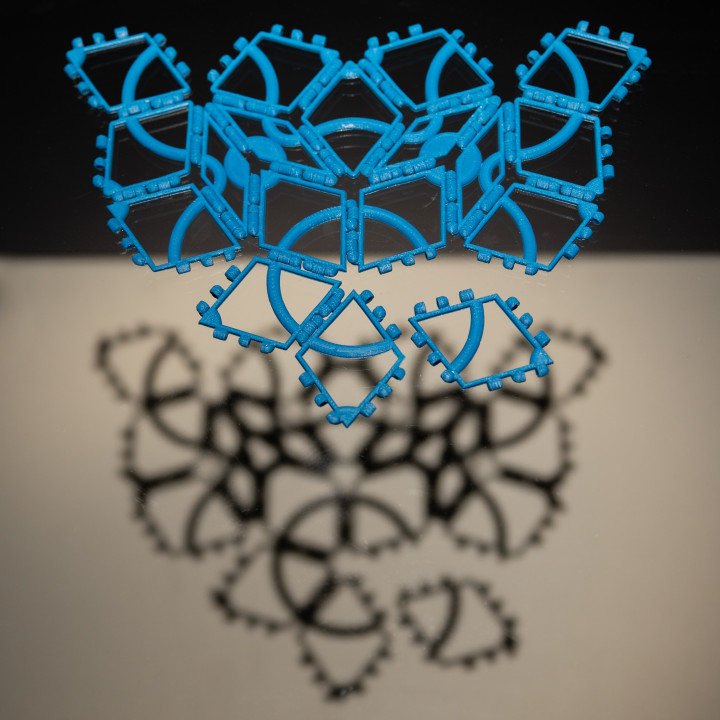
These two polypanels can be used to create a Penrose tiling pattern that never repeats no matter how many tiles are used. To achieve this, you must match up the features on the faces of the two different rhombuses like a jigsaw puzzle. Both polypanels have a segment of a curve and a segment of a filled circle on their faces that need to be matched with adjacent tiles. When the tiles are combined according to this rule, some pleasant semi-regular patterns emerge, but the pattern as a whole never repeats! This clever tiling pattern was discovered by mathematical physicist Roger Penrose. For more information about it, look at the Penrose tiling page on Wikipedia. The two rhombuses in this upload have standard polypanel side lengths but differ from the polypanel rhombus created by Devin Montes due to different internal angles. In this case, they are 72 and 108 degrees for the wider rhombus and 36 and 144 degrees for the thinner one, whereas Devin's rhombus has internal angles of 60 and 120 degrees. The 72-degree angle divides a circle five times and the 36-degree angle divides it ten times, resulting in a lot of five-fold symmetry going on in the patterns these tiles produce. Indeed, if you ignore the whole Penrose tiling aspect and just use them as a construction polypanel, they play rather nicely with pentagons. For yet another polypanel rhombus that also has interesting properties, check out my "Root Two Rhombus Polypanel". UPDATE 2019-05-20: I've added a second version of each rhombus which I think improves the visual effect of the tiling pattern. I found the gaps around the connectors in the original design were distracting away from the pattern the tiles produced. The new rhombus versions invert the design so that the hollow parts of the old versions are now solid and the solid parts are now hollow. The new versions also close most of the gaps around the connectors by adding small wings that extend outward from the base at a 45-degree angle. They need to be angled like this so as not to interfere with the polypanels being used in 3D construction models, but they still print just fine without supports. Note that you don't need as many of the thinner rhombuses as the wider ones to tile the plane, so I would recommend printing them out in a ratio of about two thinner ones for every three wider ones.