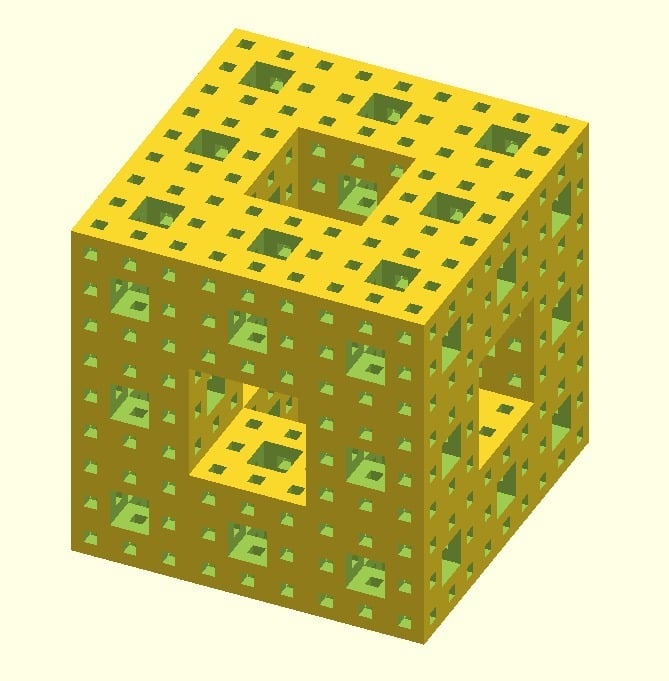
Menger sponge

thingiverse

This is a Menger sponge, an example of a three-dimensional fractal created by repeatedly removing the middle section from each face of a cube. The process starts with a standard cube and then continues with the removal of smaller cubes, resulting in a complex pattern that never fully repeats itself. The Menger sponge's unique structure is achieved through a series of iterative steps, where each step involves removing a portion of the previous iteration. This process can be repeated indefinitely, producing an infinite sequence of increasingly intricate patterns. One of the most fascinating aspects of the Menger sponge is its ability to exhibit properties that are not found in traditional geometric shapes. For instance, it has a non-integer surface area and volume, which makes it an interesting subject for mathematicians and scientists studying fractals. The Menger sponge's discovery is credited to Austrian mathematician Karl Menger, who first described the shape in 1926 as part of his work on topological spaces. Since then, the Menger sponge has become a popular topic among mathematics enthusiasts and has even inspired real-world applications such as the creation of fractal-inspired sculptures. The MegaMenger project, which is the subject of an MIT video, aims to build a life-size replica of the Menger sponge using a large number of 1-inch cubes. This ambitious project not only showcases the complexity of the Menger sponge but also demonstrates its potential for large-scale construction and display.