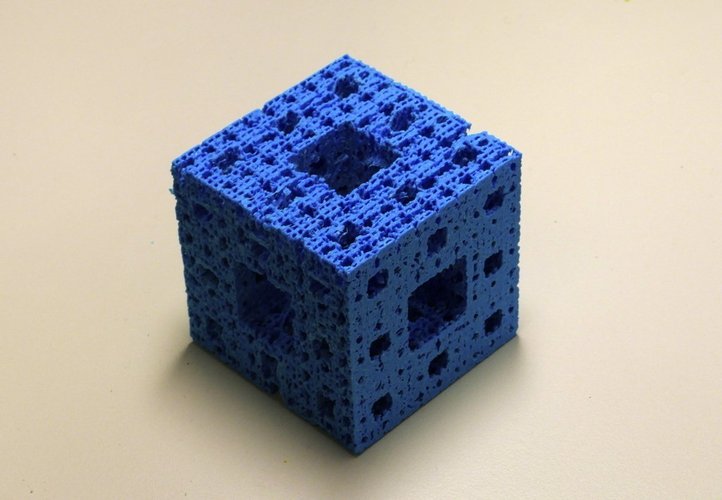
Menger's Sponge (Fractal Cube, 3D Sierpinski's Carpet)

pinshape

Summary Menger's Sponge, a three-dimensional fractal cube known as Sierpinski's carpet. This shape boasts infinite area and zero volume, making it extremely challenging to calculate in higher orders or 3D print-out. I successfully calculated up to the fourth order using my PC and third order with a cheap FDM printer. I challenge anyone to push the limits further! How I Designed This I used cube.scad for OpenSCAD to create this intricate design. This shape is infamous for its explosive calculation/memory requirements, which limited me to third-order calculations. Another method involves generating a fractal corridor using cubedig.scad, copying and rotating it in the Z->X/Y axis direction, then subtracting it from a cube through boolean difference. However, this approach was capped at fourth order due to its limitations. The "cubedig4-dig145.stl" file represents a 145mm cube corridor that's slightly longer than expected. After resizing it to one-third in Blender, I obtained the "cubedig4_48.33" result. Following these experiments, my Da Vinci 3D printer proved unable to print beyond third order due to the object's excessive fragility and low density.