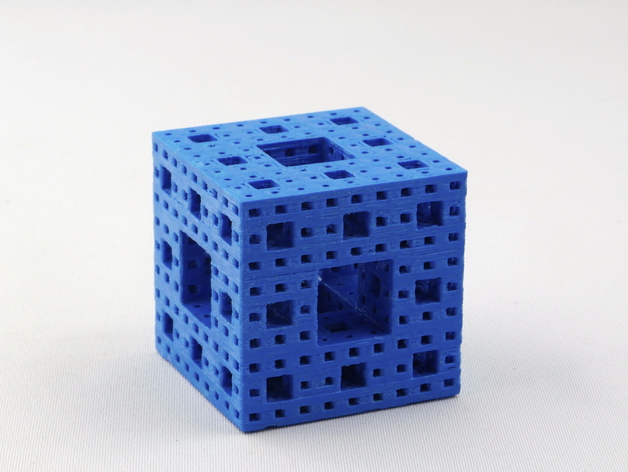
Menger's Sponge (Fractal Cube, 3D Sierpinski's Carpet)

thingiverse

Fractal cube known as Menger's Sponge or Sierpinski's carpet exhibits intriguing properties. Its area grows exponentially while its volume approaches zero, posing a formidable challenge for computational and manufacturing tasks. This shape pushes computer resources to their limits and outperforms standard 3D printing capabilities. Calculating the dimensions of higher-order Menger sponges is time-consuming and requires vast memory resources. Using high-performance PCs enabled me to reach up to 4th order calculations, while FDM printers managed a 3rd order limit. This remarkable fractal cube has inspired innovative designs due to its complex calculation demands, resulting in a unique limitation: the maximum obtainable order with 3D printing being at third level. For generating fractals like this sponge I utilized cube.scad code within OpenSCAD for rendering cube faces of any order. Menger's Sponge also benefits from the technique called 'generating a "corridor"'. Cubedig4.stl utilizes cubedig and rotation with Z -> X, then copy, to obtain this very shape; note: corridor measures more than 145mm while final resized Menger cube reaches half in size with the title: cubedig_48.33. During further refinement on 3D model printing limitations surfaced. When reaching order 4 in 2d designs - the structure simply broke because of too high density, as my printer - Da Vinci was unable print anything over third degree - objects are very fragile at higher degrees