Langford Chaotic Attractor

thingiverse

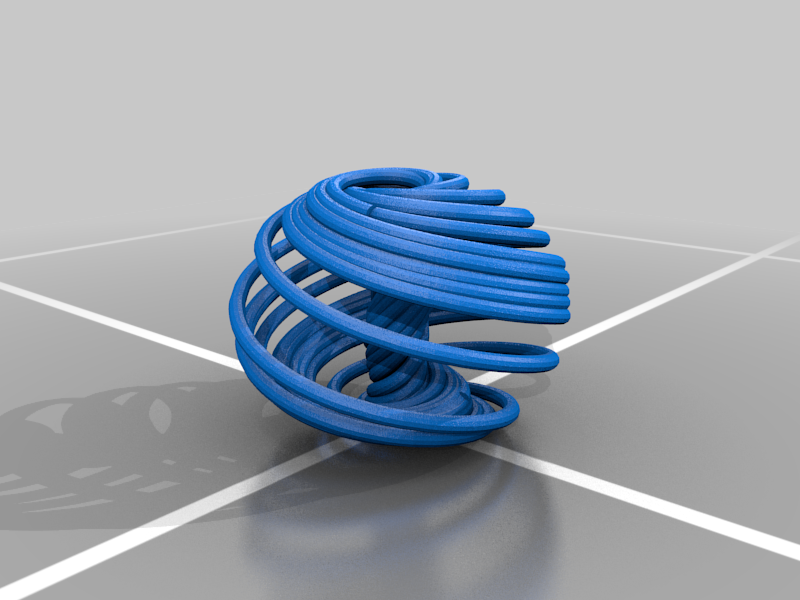
Langford Chaotic Attractor Cindy Guzman November 1st, 2021 George Mason University Math 401: Mathematics Through 3D Printing For this past week, the main topic we analyzed and discusses was the various types of strange chaotic attractors. These attracts are made up of dynamical systems structured by ordinary differential equations (ODEs). ODEs explain the relationship between a function and its derivative (Meiss). What makes these systems chaotic consists of sensitive dependency and transitivity. Out of the many strange chaotic attractors, I decided to print a Langford Chaotic attractor. This attractor was discovered from William F Langford. He wanted to come up with a model where he would produce toroidal chaos. His objective was to conduct research on bifurcations, when small changes are made to parameter values. The following ODEs were used: dx/dt=(z-b)x-dy dy/dt=dx+(z-b)y dz/dt=c+az-z^3/3-(x^2+y^2)(1+ez)+fzx^3 Unfortunately, I wasn't able to print my object myself due to printer issues. I believe my print was done through the Ultimaker. It shouldn't have taken more than 3 hours long. I played around with the initial values. I wanted to make my Langford Chaotic attracter with spaces, not almost fully solid. I didn't have to include a base since the bottom of my print was not round and could stand by itself. Lucas, Stephen, Evelyn Sander, and Laura Taalman. "Modeling Dynamical Systems for 3D Printing". 16 July 2020. Meiss, James. "Differential Dynamical Systems". Society for Industrial and Applied Mathematics, 2007.