Klein Bottle + Bagel

sketchfab

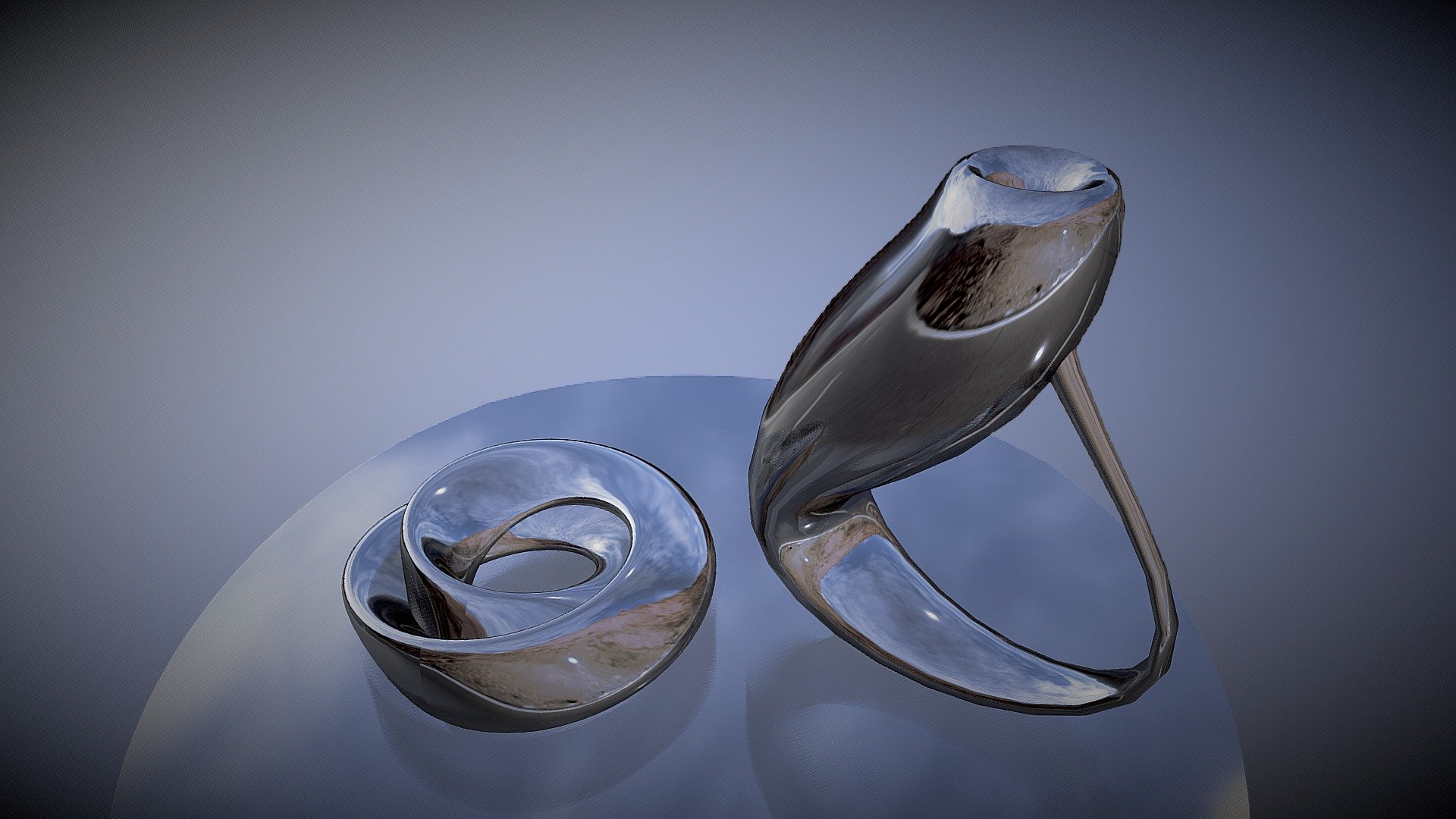
The Klein Bottle and its "Figure 8" Immersion, a Bagel-Shaped Marvel Imagine a world where geometry defies conventional norms, where a shape can be both inside and outside simultaneously. Welcome to the realm of the Klein bottle, a mind-bending mathematical concept that has fascinated mathematicians and scientists for centuries. The Klein bottle is named after Felix Klein, a German mathematician who first described it in the late 19th century. It's a two-dimensional surface that can be embedded in three-dimensional space, but with a twist - it has no edges or boundaries. Instead, it wraps around itself in a continuous loop, creating a seamless union of inside and outside. But what's truly remarkable about the Klein bottle is its "figure 8" or "bagel" immersion, where it takes on a torus-like shape. This is achieved by embedding the Klein bottle in a four-dimensional space, allowing it to twist and turn in ways that would be impossible in lower dimensions. The equations governing this phenomenon are quite simple, yet profound. They involve the use of complex numbers and differential geometry, but don't worry if you're not a math whiz - the beauty of the Klein bottle lies in its ability to inspire and fascinate, regardless of your mathematical background. For a deeper dive into the world of the Klein bottle, check out the Wikipedia article on its parametrization. This will give you a more detailed understanding of the equations and how they relate to this fascinating shape. As you explore the Klein bottle, remember that it's not just a abstract concept - it has real-world applications in fields like topology, geometry, and physics. It's a reminder that math is all around us, waiting to be discovered and explored.