Hyperbolic Paraboloid

thingiverse

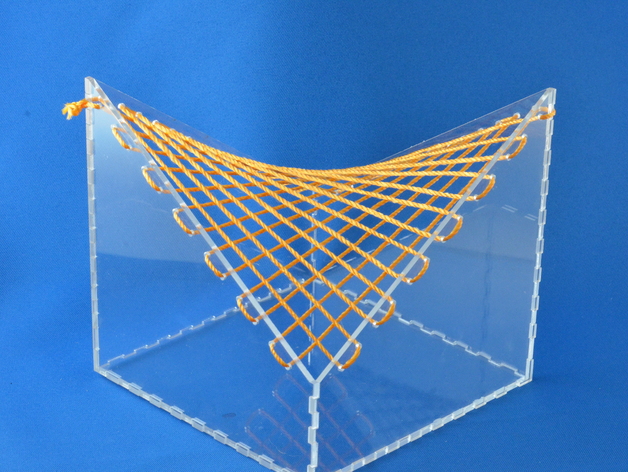
A hyperbolic paraboloid, a quadric surface defined by z = y^2/a^2 - x^2/b^2, features a saddle point at its center, where it curves upwards and downwards simultaneously. Leveraging their doubly ruled nature, wherein each point has two lines passing through it on the quadric surface, I created this object using straight lines represented by string. This seemingly complex design arises from simple rules and was made with an Epilog Mini 18 Laser, cutting 3mm acrylic for both sides, glued together with acrylic glue. The string opening is 1/10in., which accommodates standard strings but may need adjustment for thicker varieties.