Fubini's Theorem Lesson

thingiverse

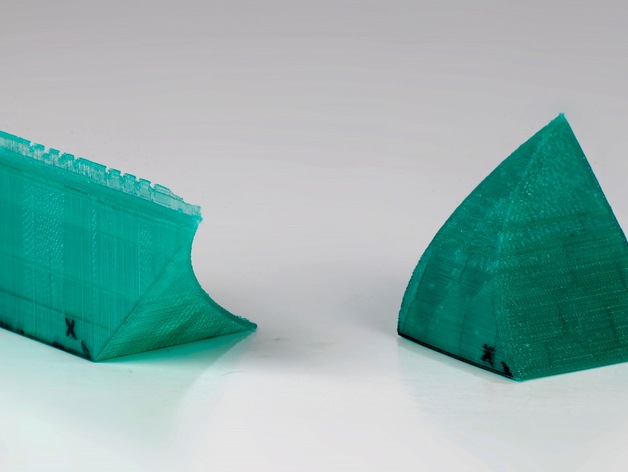
These two distinct shapes, Region E and Region F, are designed to be a crucial part of a multivariable calculus lesson on three-dimensional solid regions and the principles of Fubini's Theorem and Cavalieri's Principle. Students receive two sets of inequalities below and must first determine which model corresponds to each set of inequalities. Inequalities 1 Inequalities 2 0 < x < 1-z 0 < x < z+1 z^2 < y < 1 0 < y < (z-1)^2 0 < z 0 < z Students then use calculus to compare the volumes of the two shapes. This page is designed to complement a paper titled "3D Printed Manipulatives in a Multivariable Calculus Classroom" that will be published in PRIMUS. I used Mathematica's RegionPlot3D function to design these shapes. Specifically, for the first set of inequalities, RegionPlot3D[ x == z^2, {x, 0, 1}, {y, 0, 1}, {z, 0, 1}, BoxRatios -> Automatic, AxesLabel -> Automatic, PlotPoints -> 100 ] For the second set of inequalities, I used RegionPlot3D[ x < (1 - z)^2, {x, 0, 1}, {y, 0, 1}, {z, 0, 1}, BoxRatios -> Automatic, AxesLabel -> Automatic, PlotPoints -> 100 ] These generated the following solid regions in Mathematica, which were exported to STL files using the Export function. I scaled the shapes so that the unit length was 3 inches. Project: Solid Regions and Fubini's Theorem Overview & Background One of the primary themes in multivariable calculus is finding the volume of three-dimensional solid regions. To begin this topic, we must establish a language for describing three-dimensional figures. One way to do this is by using inequalities in the three coordinate variables x, y, and z. The first part of this lesson gives students practice with interpreting a set of inequalities as a description of a solid region. To start volume calculations, students use Fubini's Theorem, which tells us that the volume of a solid is equal to the definite integral of the cross-sectional area function. In other words, we can imagine cutting each figure into thin slices, multiplying the cross-sectional area of each slice by the thickness (estimating the volume of each slice), and then adding up the results to estimate the total volume. The thinner the slices, the better the estimate, and in the limit (with "infinitely thin" slices), we get the exact volume. This process is also known as Cavalieri's Principle. Lesson This lesson is best conducted in groups of two to four students. I printed a copy of each shape for every group, and I drew coordinate axes on the shapes with a Sharpie. Once groups were decided, each group was given the labeled shapes of Region E and Region F, and told to answer the following questions. Below are two sets of inequalities. Which set of inequalities corresponds to Region E, and which corresponds to Region F? Give reasons for your answer. Inequalities 1 Inequalities 2 0 < x < 1-z 0 < x < z+1 z^2 < y < 1 0 < y < (z-1)^2 0 < z 0 < z Sketch cross-sections of each solid region taken parallel to the xy-plane. What shape are the cross-sections? Find the areas of the cross-sections as functions of z. Which region has a larger volume, E or F? Explain your answer in a few sentences. Preparation It is essential that every student has a chance to handle the shapes, so I made sure to print enough copies of the shapes ahead of time. I also think it was helpful to draw coordinate axes directly onto the shapes so that students could orient them correctly. As a warm-up to this activity, I had students deal with a simpler set of inequalities. In particular, I asked them to take 10 minutes at the beginning of class to build out of Play-Doh the region described by: 0 < x < 1 0 < y < 1 0 < z < x+y I found that this primed students with some strategies for thinking about how inequalities relate to solid regions.