Flamingo PG Wallpaper Tiles

thingiverse

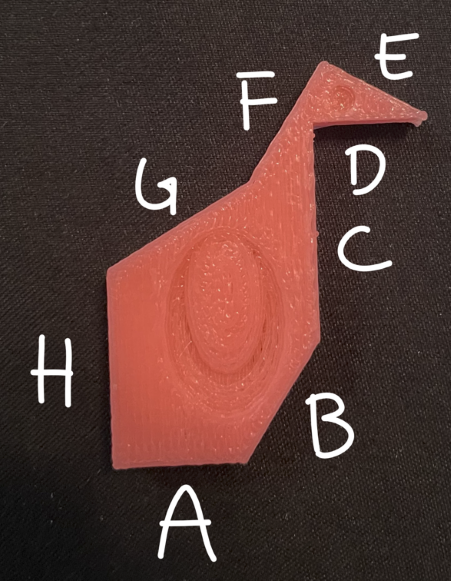
George Mason University Math 401: Mathematics Through 3D Printing 09/20/2021 Marlanna Bozicevich Background Seventeen wallpaper groups were classified in the late 19th century by Russian mathematician Evgraf Stepanovich Fedorov, German mathematician Arthur Moritz Schoenflies, and English mathematician William Barlow. Any repeated pattern on a 2D plane can be classified into one of these 17 groupings. These groups have a variety of sub-features including lattice structure, rotation count, glide reflections, mirroring lines, and traditional reflections. My piece is an example of a PG wallpaper tiling, one of the most simplistic groups out of the 17 different types. In this group, there are no rotations nor reflections with a rectangular lattice structure. The essential characteristic in this group is glide reflections. The direction of the glide reflection is parallel to one group of translated tiles and perpendicular to the adjacent one, meaning that the same tile pattern is repeated every other "column." The backside of my tiles versus the designed front side hopefully allows distinction of these glide translations. Code Explanation The creation of the tile shape was quite simple if you understand which side length and slopes need to be equivalent. When looking at the labeled photograph of the 3D printed tile, the following had to be true to tile a surface: A = D, B = F, C = H, E = G. Additionally, the slopes of B and F must be equivalent, while the slopes of E and G must be negative equivalent. Right angles are necessary between sides A and H, as well as between sides C and D. I connected these points with the polygon() command combined with linear extrude in openSCAD. The details of my abstract “flamingos” were added with the difference() command. Lastly, note that the command offset(delta=-0.5) allows the tiles to properly fit with one another once printed. Without this setting, the tiles would be mathematically correct, but the error of printing would not allow for a perfect fit from tile to tile. Printing Details At the time of printing, scaling was done to control the size using the MakerBot software. I adjusted in the x and y direction uniformly but kept the z-direction the same so that the height of each tile would remain around 5mm. I changed the x and y dimensions so that the longest length of the tile was about 50mm. The openSCAD code only contained one tile therefore duplicates were also made right before printing. I adjusted them to be as close as possible to save space and time. All eight tiles took approximately three and a half hours to print, including a raft to keep the tiles connected. The MakerBot Replicator printed this piece. Citations https://www2.clarku.edu/faculty/djoyce/wallpaper/seventeen.html https://www2.clarku.edu/faculty/djoyce/wallpaper/history.html https://cpb-us-w2.wpmucdn.com/sites.uwm.edu/dist/0/158/files/2017/04/Liu-Collins.Frieze-and-wallpaper-symmetry-groups.Classification-under-affine-and-perspective-distortion.1999-21luqq4.pdf https://www.bemidjistate.edu/academics/honors/wp-content/uploads/sites/73/2017/03/Wallpaper-Groups-Fett-Cassondra.pdf