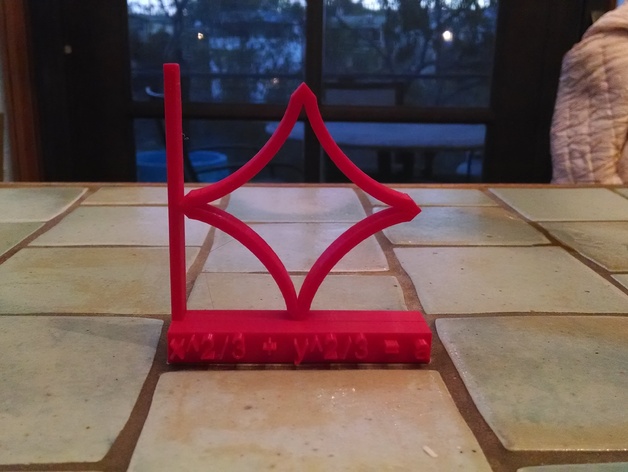
Daily Equation #2 - Astroid x^(2/3) + y^(2/3) = a

thingiverse

On day 2 of my collection of printable equations, the astroid is featured. Discovered by Johann Bernoulli in 1691-92, it's also known as the tetracuspid for its four cusps. The length of an astroid is 6r and its area 3(r^2)/8. It can be formed by rolling a circle of radius r/4 on the inside of a circle of radius r or by moving a line segment with each end on perpendicular axes, making it a glissette. The astroid only acquired its current name in 1836. It's been known as the cubocycloid and paracycle in various literature sources. The provided STL file has units in mm, 'a' set at 70, and range of x from -50 to 50, while maintaining a 100mm x-axis. As always, one equation will be posted daily until interest wanes or suggestions are made for specific equations. Note that artifacts may occur in regions of rapidly changing slope when plotting the second curve perpendicular to the first's tangent, but the lower or inner curve remains accurate. Printing without support is possible for all files; photos were taken using a Replicator 2 and Makerware with default settings.