stlfinder - 3d models search engine stlfinder - 3d models search engine
Contour Lines for z = e^(-(x^2 + y^2))

Contour Lines for z = e^(-(x^2 + y^2))

thingiverse

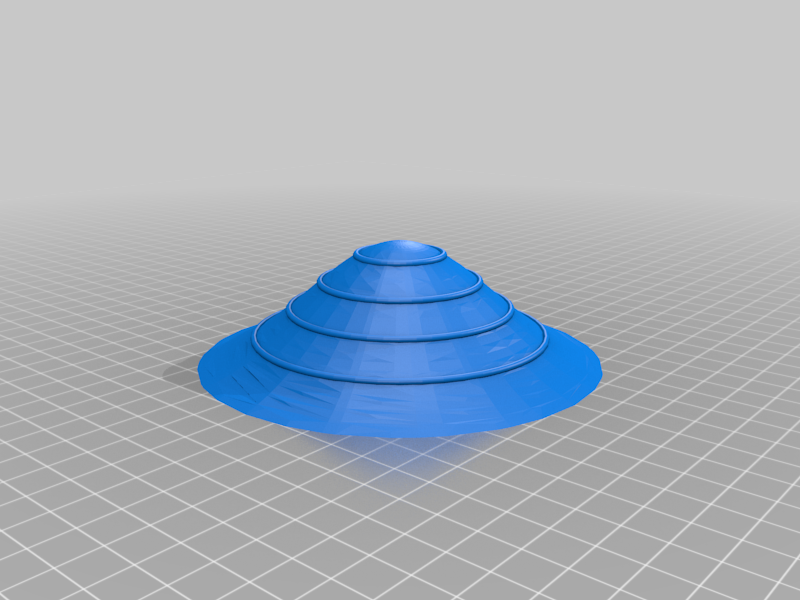
Contour Lines for z = e^(-(x^2 + y^2)) Ryan Simmons 11/8/2021 George Mason University Math 401 The chosen function for this print is z = e^(-(x^2 + y^2)). This function’s domain is (0,1]. Since this function will never reach 0, it is printed starting at z=.1 instead. The rings on the print are contour curves. Contour curves are the intersection of the surface and the xy plane at some z value. This print shows contour curves at z=.3, z=.5, z=.7, and z=.9.

Download Model from thingiverse

With this file you will be able to print Contour Lines for z = e^(-(x^2 + y^2)) with your 3D printer. Click on the button and save the file on your computer to work, edit or customize your design. You can also find more 3D designs for printers on Contour Lines for z = e^(-(x^2 + y^2)).