Chaotic Attractor

thingiverse

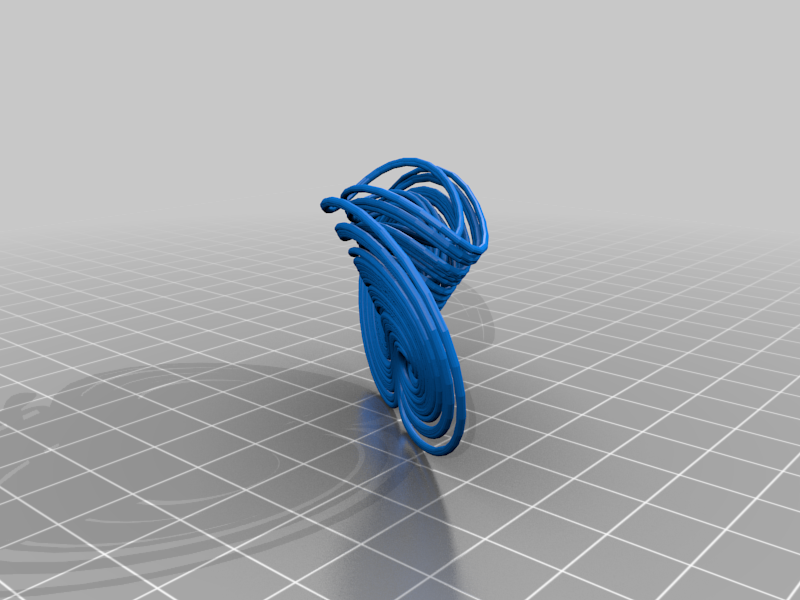
Chaotic Attractor by Gabriela Castaneda Guzman 11/02/2022 George Mason University Math 401: Mathematics Through 3D Printing The print was successfully rendered on Wolfram Mathematica, and sliced through Ultimaker Cura to print on 2-filament 3D printer one being water-soluble (for support). Object was printed alongside 2 more chaotic attractors bringing the time to around 30-hour print, after rotating to reduce amount of support needed. Was later floating in water for 2.5 days after which most water-soluble filament was gone. A chaotic or strange attractors are complex dynamical systems that show a particular sensitivity to initial conditions. As shown in code below, the initial conditions for this attractor are a = 35, b = 3, and c = 28. These initial conditions were employed as explained in “Modeling Dynamical Systems for 3D Printing” by Lucas, Sander, and Taalman. Under these conditions, this attractor has been dubbed “yet another chaotic attractor” a variation of the Lorenz system of equations. Thickness of the object’s tube can be changed according to what individual 3D printers are apt for. Using water-soluble filament for the object’s support made it possible for the object’s tubes to be on the thinner side. As shown on the post print section, print needed much cleaning/polishing post print but still conserved the integrity of the thin tubes.