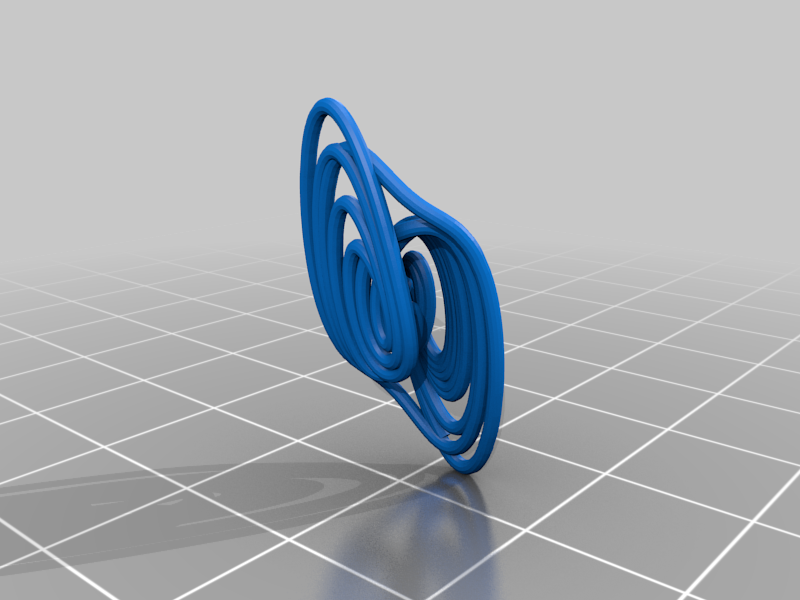
Chaotic Attractor

thingiverse

The object is the Arneodo chaotic attractor. The Mathematica code attached can be used to produce a variety of chaotic attractors by changing the constant parameters, initial conditions, and functions F1, F2, and F3. Chaotic attractors demonstrate the instabilities we encounter in nature and everyday life. In moments of idleness in class or at the office, in simple pastimes like balancing a pencil vertically on a table we witness the inherent chaos in the universe. The study of chaos studies sensitive systems, ones such that a small change in parameters can cause large changes in the system. A system can change from converging onto a solution into changing erratically. This is what is called chaos. That is why no matter how carefully you balance your pencil on the table, it doesn't stay. The slightest disruption causes massive shifts in the system. The phrase "the butterfly effect" rose from this question of sensitivity and chaos in the universe. If the weather system is sensitive, is it possible for the flap of a butterfly's wing would cause a hurricane across the globe? Or is it possible the next slight increase in global temperatures causes massive effects? The study of chaos gives insight into these possibilities. The object was made by solving for the coordinates of a system defined by it's partial derivatives. The first part of the code starts the system from an arbitrary points. At the end of that point's path, the system continues in the second half of the code. This first section removes the "start up" of the system, called removing transients. This lets the system converge to the attractor shape, which makes the final attractor look better. For printing the object, I printed the object sideways, with it's longest side as the height. This reduced the number of support contact points, letting the top and bottom surfaces of the attractor appear smoother and cleaner. It also made the supports easier to remove from inside the attractor. Alternatively, if you have access to it, dissolvable supports would make the process of printing a chaotic attractor easier, especially for other types of attractors with hollow parts. The object itself shows a single chaotic system, but playing with the code gives more insight into chaotic and sensitive systems. Modifying the parameters very slight can show when the system becomes chaotic. For example, in example in the code, around a = -3.8, as the value of a slowly decreases, the system becomes chaotic. Additionally, if you modify F1,F2, and F3 using partial derivatives for another attractor, this same code process can be used to create many different attractors.