Bihelicoid in S^3

sketchfab

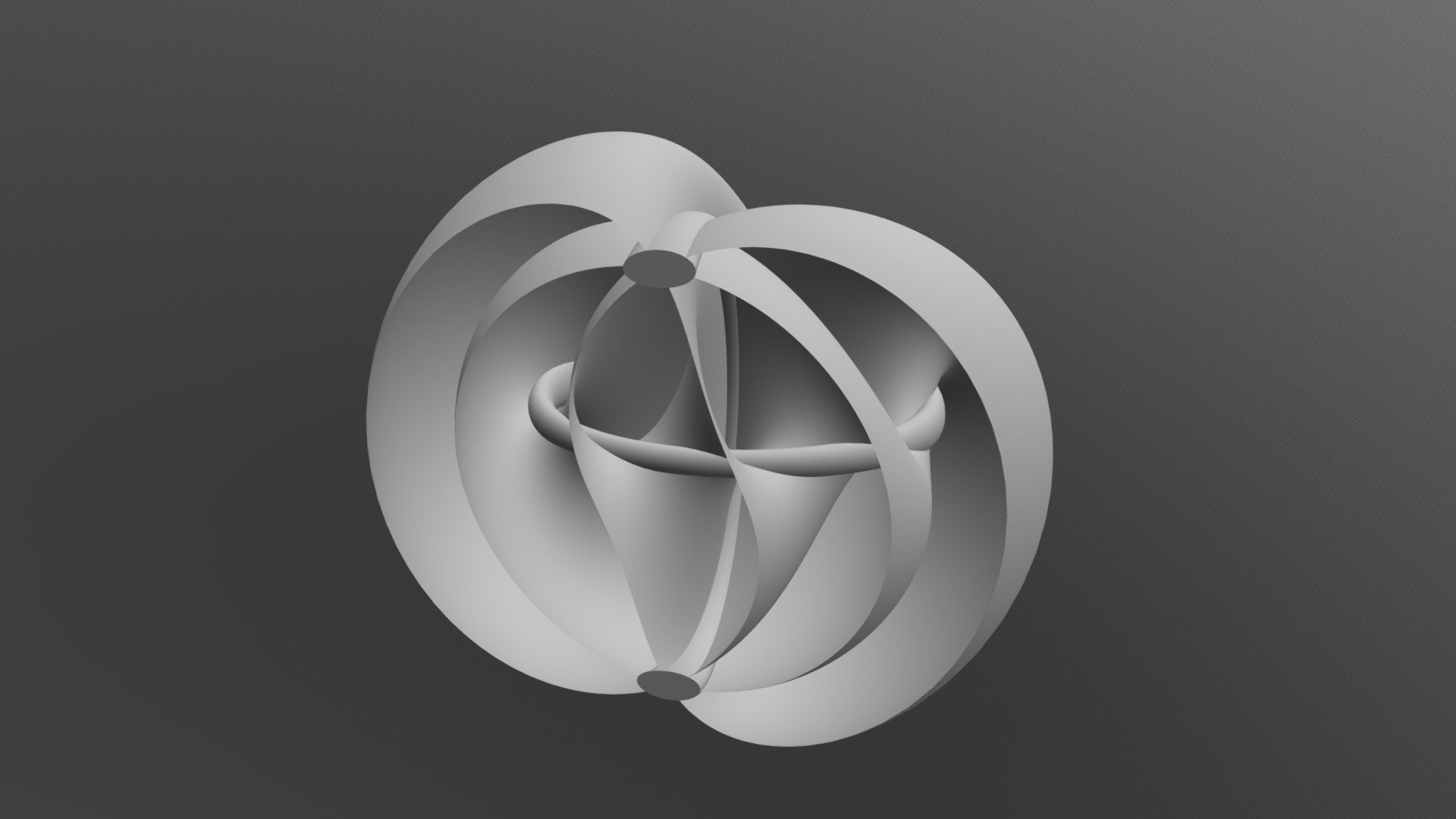
This is a minimal surface described in the paper "Minimal submanifolds of the bicylinder boundary" by Thomas Banchoff. Specifically, it's a bihelicoid from section 2 with parameters m=3 and n=1. This surface is an immersion of a torus with incident points along one axis of the defining bicylinder. We rotated the surface to project its linked axes symmetrically onto R^3. The projection itself is an azimuthal equidistant projection, mapping S^3 to a ball. To view the interior, we cut away part of the surface.